Cubic feet are a unit of volume commonly used to measure the capacity of three-dimensional objects or spaces. It is derived from the measurement of length in feet and represents the volume of a cube with sides that are each one-foot long.
Cubic feet are frequently used in various fields, including construction, architecture, engineering, and everyday measurements. It is commonly used to quantify the volume of objects, such as rooms, buildings, containers, or any three-dimensional space. For example, the volume of a storage box or the capacity of a swimming pool may be measured in cubic feet.
In this article, we will discuss the concept of cubic feet, the formulas of cubic feet, and the application of cubic feet. Also, the topic will be explained with the help of a detailed example.
What are cubic feet?
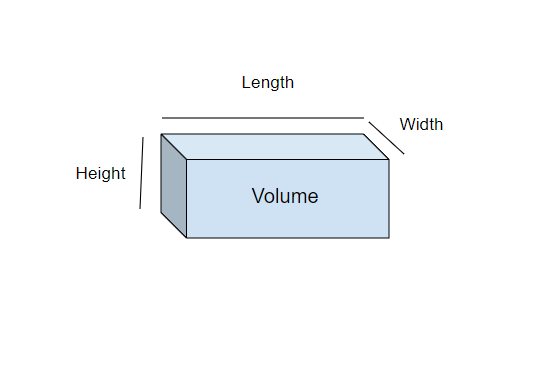
Cubic feet are a unit of volume that measures the three-dimensional space occupied by an object or an enclosed area. It is derived from the measurement of length in feet and represents the volume of a cube with sides that are each one-foot long. The term “cubic” refers to the fact that it involves three dimensions: length, width, and height.
The term “cubic” in cubic feet indicates that it involves three dimensions: length, width, and height. When these dimensions are multiplied together, the result is expressed in cubic units, such as cubic feet.
Formulas of cubic feet
Cubic Feet of a Rectangular Prism:
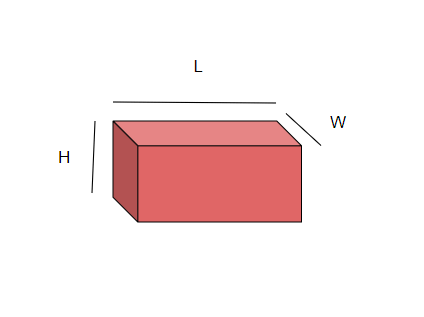
If you have the measurements of the length, width, and height of a rectangular prism, you can calculate its volume (in cubic feet) using the formula:
Volume = Length × Width × Height
Cubic Feet of a Cylinder:
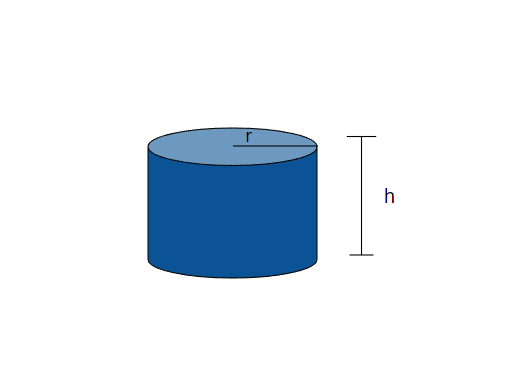
If you have the measurements of the radius (r) and height (h) of a cylinder, you can calculate its volume (in cubic feet) using the formula:
Volume = π × r² × h
Note: π is the constant and the value of pi= 3.14159.
Cubic Feet of a Cone:
If you have the measurements of the radius (r) and height (h) of a cone, you can calculate its volume (in cubic feet) using the formula:
Volume = (π × r² × h) / 3
Cubic Feet of a Sphere:
If you have the measurement of the radius (r) of a sphere, you can calculate its volume (in cubic feet) using the formula:
Volume = (4/3) × π × r³
Remember to ensure that all measurements are in the same unit (e.g., feet) before using these formulas to calculate the cubic
Applications of Cubic Feet:
Cubic feet are a unit of volume commonly used in various applications. Now, we discuss some basic applications of cubic feet.
Construction and Architecture: Cubic feet are used extensively in construction and architecture to calculate the volume of various elements such as rooms, buildings, concrete pours, and construction materials. It helps in determining the required number of materials like concrete, paint, or flooring for a given project.
Shipping and Freight: Cubic feet are used in the transportation industry, particularly for determining the capacity and volume of shipping containers, trucks, and cargo holds. It helps in estimating the space required to transport goods and optimizing the loading and unloading process.
Storage and Warehousing: Cubic feet are used to measure the capacity of storage units, warehouses, and containers. It helps in determining how much space is available and how much can be stored. It is also used in calculating storage costs and optimizing storage layouts.
Packaging and Shipping Products: Cubic feet are used to determine the size and volume of packaging for products. It is essential for logistics and shipping companies to ensure that products fit into appropriate boxes or containers and to calculate shipping costs based on volumetric weight.
HVAC (Heating, Ventilation, and Air Conditioning): Cubic feet are used in HVAC systems to determine the volume of space for proper air conditioning, heating, and ventilation. It helps in sizing HVAC equipment and designing efficient systems.
Aquariums and Fish Tanks: Cubic feet are used to measure the volume of aquariums and fish tanks. It helps in determining the appropriate amount of water required, selecting the right filtration system, and calculating the space needed for fish and other aquatic animals.
These are just a few examples, but cubic feet are a versatile unit of volume that finds application in various fields where measuring volume is necessary.
How to Find Cubic Feet?
Example 1:
Calculate the cubic feet of the given measurements of a room.
Length = 13ft, Width = 6ft, and Height = 17ft
Solution:
Given data is
Length = 13ft
Width = 6ft
Height = 17ft
Step 1:
The formula for cubic feet
Cubic Feet = Length (ft) × Width (ft) × Height (ft)
Step 2:
Now put the above-given value
Cubic Feet = 13 × 6 × 17
Cubic Feet = 1326 ft3
Example 2:
Calculate cubic feet of the given measurements of the tank.
Length = 4yd, width = 20yd, and height = 31yd
Solution:
Given data
length = 4 yd
width = 20 yd
height = 31 yd
Step 1:
Formula
Cubic Feet = Length (ft) × Width (ft) × Height (ft)
First, we convert all (yd) units into feet
4 yd = 3×4 = 12 ft
20 yd = 60 ft
31 yd = 93 ft
The values after conversion are as:
length = 12 ft, width = 60 ft, height = 93 ft
Step 2:
Now we have to put values in the above formula:
Cubic Feet = 12 × 60 × 93
Cubic Feet = 66960 ft3
Using a cubic feet calculator is an easy way to find the volume of different object in cubic feet with steps.
FAQs
FAQs
Tell the many cubic feet on one cubic meter.
There are approximately 35.3147 cubic feet in one cubic meter. To convert from cubic meters to cubic feet, multiply the number of cubic meters by 35.3147.
How can I convert cubic feet to gallons?
The conversion from cubic feet to gallons depends on the type of gallon used. In the US customary system, there are 7.48052 gallons in one cubic foot. So, when we convert the cubic feet into gallons, we multiply the number into 7.48052.
How can I estimate cubic feet for irregularly shaped objects or spaces?
For irregularly shaped objects or spaces, you can use various techniques to estimate cubic feet. One common method is to divide the object or space into smaller regular shapes (such as cubes or rectangular prisms), measure their dimensions, and calculate the volume of each shape. Then, sum up the volumes to get an estimate of the total cubic feet.
Conclusion
In this article, we have discussed the concept of cubic feet, the formulas of cubic feet, and the application of cubic feet. In addition, the topic will be explained with the help of detailed examples. After completely understanding this article, anyone can defend this topic easily.
Read Also: